Table of Links
4 Members of Deep Π0 1 classes
2. Background
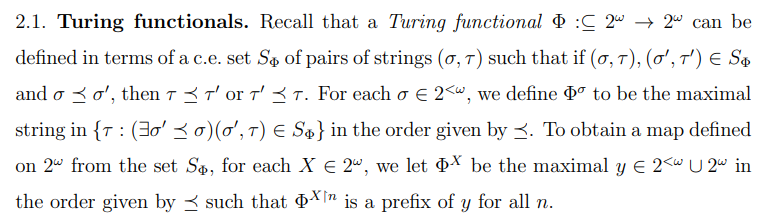
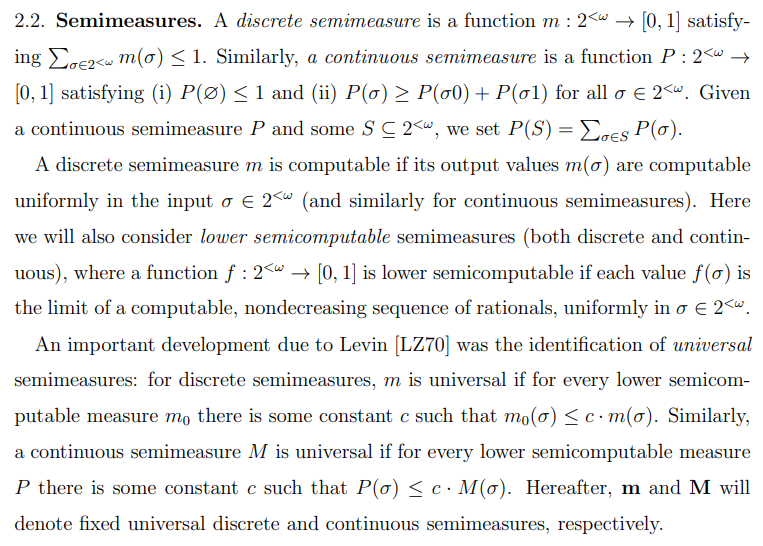
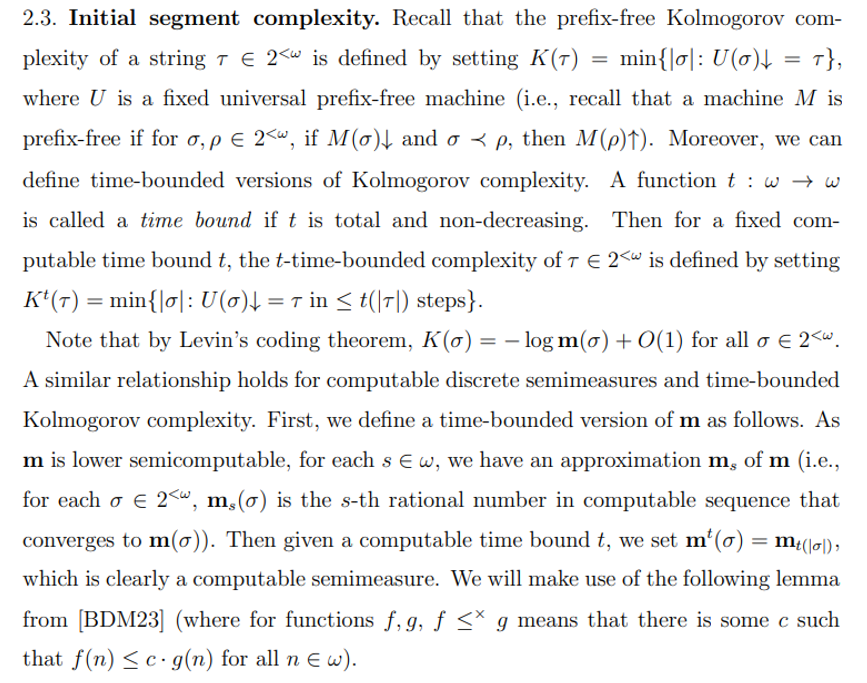
Lemma 1 ([BDM23]).

In addition, we need the following theorem (see, e.g. [JLL94, Theorem 4.3(2)]).
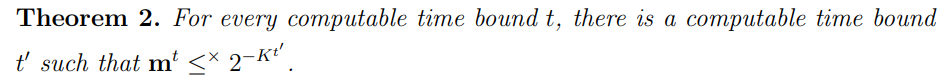
Note that by combining Lemma 1(iii) and Theorem 2, we obtain a resource-bounded analogue of Levin’s coding theorem.
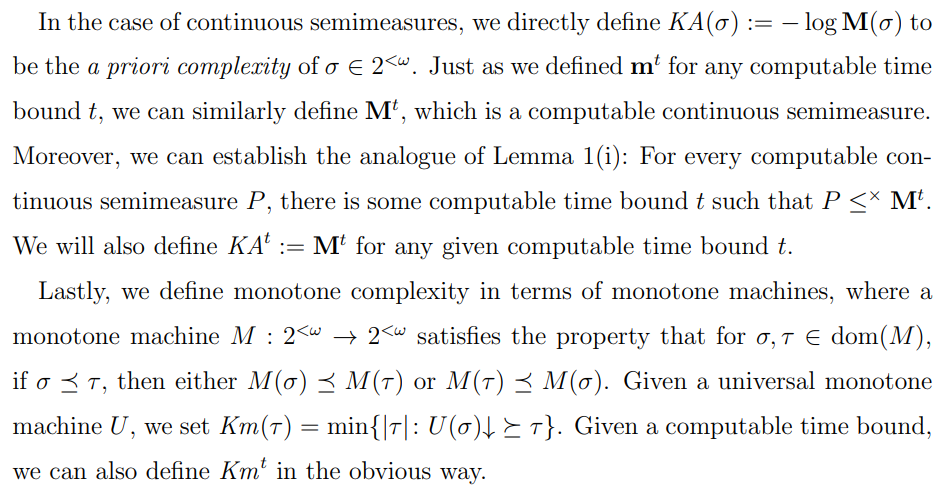
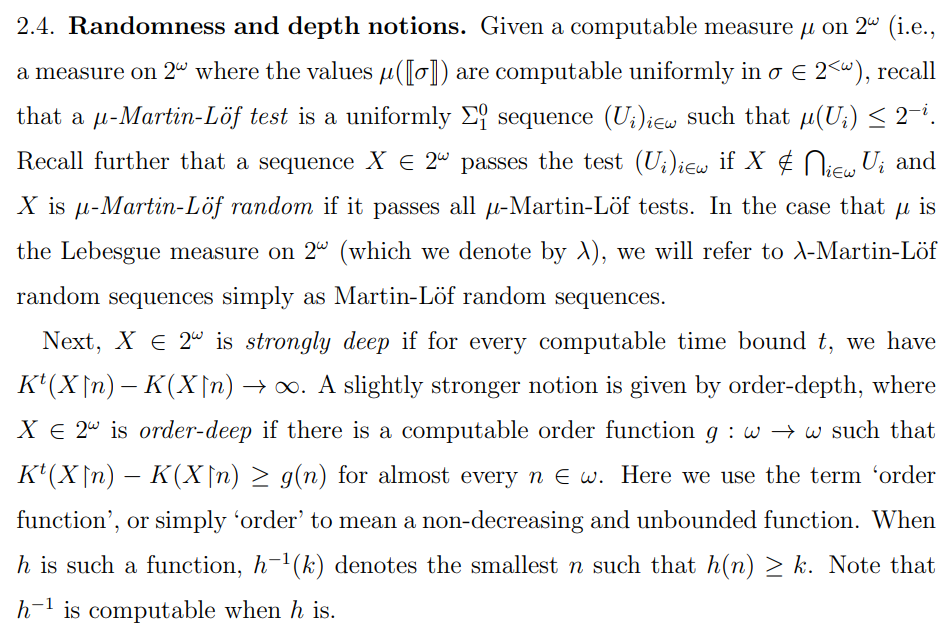
In the rest of the paper we will sometimes use an equivalent characterization of order-depth, given by the following lemma.

The proof of this lemma is technical; for the sake of readability, we defer it to the appendix.

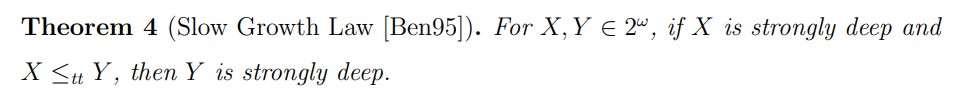
The slow growth law also holds for order-depth.
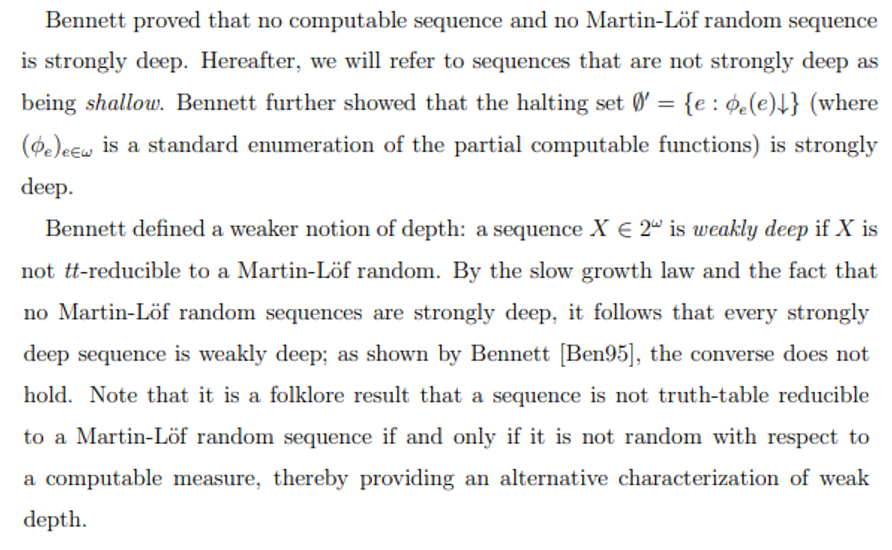

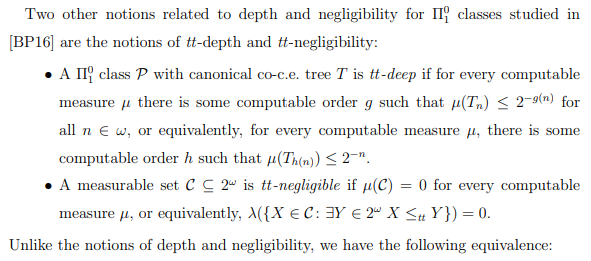
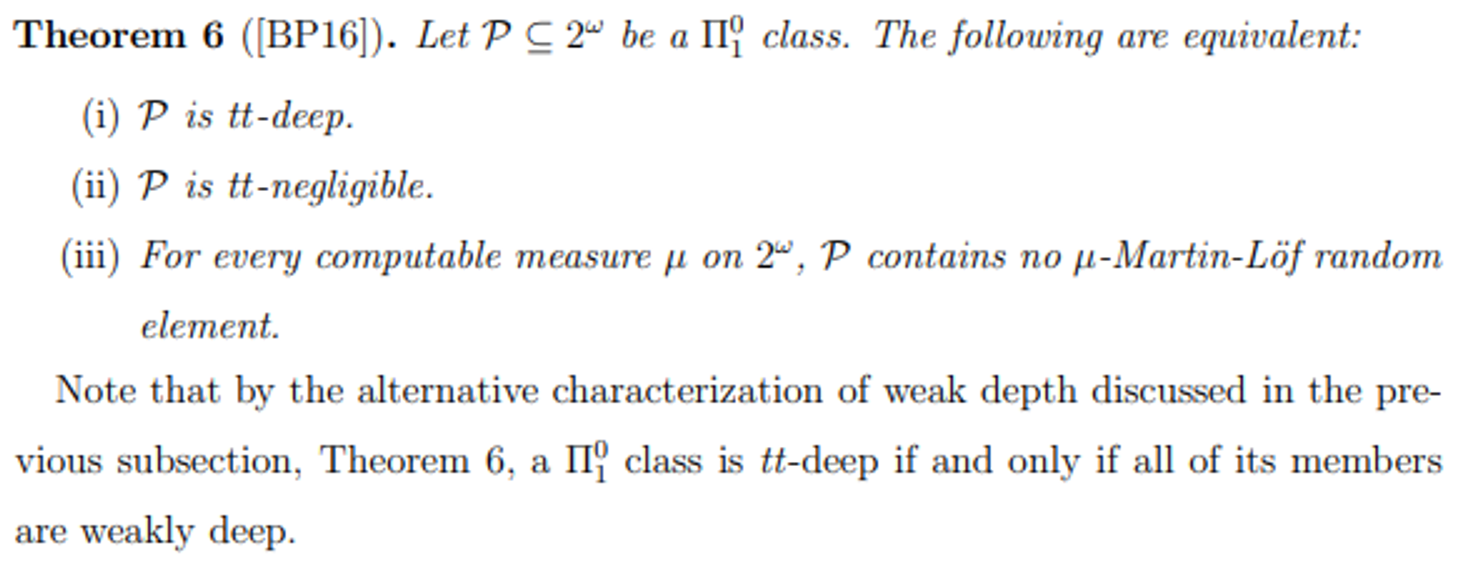
This paper is available on arxiv under CC BY 4.0 DEED license.
Authors:
(1) Laurent Bienvenu;
(2) Christopher P. Porter.